On Graph Invariants of Oxide Network

1. Introduction

Many studies have shown that there is a strong intrinsic link between the chemical properties of chemical compounds and drugs (such as boiling point and melting point) and their molecular structure. The topological index defined on the structure of these chemical molecules can help researchers better understand the physical characteristics, chemical reactivity and biological activity. Therefore, the study of topological indices of chemical substances and chemical structures of drugs can make up for the lack of chemical experiments and provide theoretical basis for the preparation of drugs and chemical substances.

In the past two decades, a large number of graph invariants (topological indices) have been defined and used for correlation analysis in theoretical chemistry, pharmacology, toxicology and environmental chemistry.

The first and second Zagreb indices are one of the oldest and most well-known topological indices defined by Gutman in 1972 and are given different names in the literature, such as the Zagreb group index, Sag. Loeb group parameters and the most common Zagreb index. The Zagreb index is one of the first indices introduced and has been used to study molecular complexity, chirality, ZE isomers and heterogeneous systems. The Zagreb index shows the potential applicability of deriving multiple linear regression models.

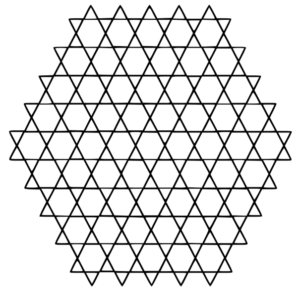
The first and the second Zagreb indices [1] are defined as \begin{equation*} M_{1}(G)=\prod\limits_{u\in E(G)}(d_{u}+d_{v}), \end{equation*} \begin{equation*} M_{2}(G)=\prod\limits_{uv\in E(G)}d_{u}\times d_{u}. \end{equation*} For details see [2]. Considering the Zagreb indices, Fath-Tabar ([3]) defined first and the second Zagreb polynomials as $$M_{1}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}+d_{v}}$$ and $$M_{2}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}.d_{v}}$$ The properties of \(M_{1}(G,x)\) and \(M_{2}(G,x)\) for some chemical structures have been studied in the literature [4, 5]. After that, in [6], the authors defined the third Zagreb index $$M_{3}(G)=\sum\limits_{uv\in E(G)}(d_{u}-d_{v}),$$ and the polynomial $$M_{3}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}-d_{v}}.$$ In the year 2016, [7] following Zagreb type polynomials were defined $$M_{4}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}(d_{u}+d_{v})},$$ $$M_{5}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{v}(d_{u}+d_{v})},$$ $$M_{a,b}(G,x)=\sum\limits_{uv\in E(G)}x^{ad_{u}+bd_{v}},$$ $$M'_{a,b}(G,x)=\sum\limits_{uv\in E(G)}x^{(d_{u}+a)(d_{v}+b)}.$$ Ranjini et al. [8] redefined the Zagreb indices, i.e, the redefined first, second and third Zagreb indices of graph \(G\). These indicators appear as $$Re ZG_{1}(G)=\sum\limits_{uv\in E(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}},$$ $$Re ZG_{2}(G)=\sum\limits_{uv\in E(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}},$$ and $$Re ZG_{3}(G)=\sum\limits_{uv\in E(G)}(d_{u}+d_{v})(d_{u}.d_{v}).$$ For details about topological indices and its applications we refer [9, 10, 11, 12, 13, 14, 15, 16, 17]. In this paper we aim to compute Zagreb polynomials and redefined Zagreb indices of Oxide networks shown in Figure 1.

2. Main Results

In this section we give our main computational results.

In this section, we present our computational results.

Theorem 2.1. Let \(OX_{n}\) be the Oxide network. Then

1. \(M_{3}(OX_{n},x)=12nx^{2}+(18n^{2}-12),\)
2. \(M_{4}(OX_{n},x)= 12nx^{12}+(18n^{2}-12)x^{32},\)
3. \(M_{5}(OX_{n},x)=12nx^{24}+(18n^{2}-12)x^{32},\)
4. \(M_{a,b}(OX_{n},x)=12nx^{2a+4b}+(18n^{2}-12)x^{4(a+b)},\)
5. \(M'_{a,b}(OX_{n},x)=12nx^{(2+a)(4+b)}+(18n^{2}-12)x^{(4+a)(4+b)}\).

Proof. Let \(OX_{n}\) be the oxide network. It is clear that \(OX_{n}\) has two partitions of vertex set i.e, \(V_{1}=\{v\in V(OX_{n}): d_{v}=2\}\) and \(V_{2}=\{v\in V(OX_{n}): d_{v}=4\}\). The edge set of \(OX_{n}\) has following two partitions, $$E_{1}=E_{2,4}=\{e=uv\in E(OX_{n}): d_{u}=2, d_{v}=4\},$$ $$E_{1}=E_{4,4}=\{e=uv\in E(OX_{n}): d_{u}=4, d_{v}=4\}.$$ Such that $$\mid E_{1}(OX_{n})\mid=12n,$$ $$\mid E_{2}(OX_{n})\mid=18n^{2}-12n.$$

1. \begin{eqnarray*} M_{3}(OX_{n},x)&=& \sum\limits_{uv\in E(G)}x^{d_{u}-d_{v}}\\ &=&\sum\limits_{uv\in E_{1}(OX_{n})}x^{4-2}+\sum\limits_{uv\in E_{2}(OX_{n})}x^{4-4}\\ &=&\mid E_{2}(OX_{n})\mid x^{2}+\mid E_{2}(OX_{n})\mid \\ &=& 12nx^{2}+\left(18n^2-12n\right). \end{eqnarray*} 2. \begin{eqnarray*} M_{4}(OX_{n},x)&=& \sum\limits_{uv\in E(OX_{n})}x^{d_{u}(d_{u}+d_{v})}\\ &=&\sum\limits_{uv\in E_{1}(OX_{n})}x^{2(2+4)}+\sum\limits_{uv\in E_{2}(OX_{n})}x^{4(4+4)}\\ &=&\mid E_{2}(OX_{n})\mid x^{12}+\mid E_{2}(OX_{n})\mid x^{32}\\ &=& 12nx^{12}+\left(18n^2-12n\right)x^{32}. \end{eqnarray*} 3. \begin{eqnarray*} M_{5}(OX_{n},x)&=& \sum\limits_{uv\in E(OX_{n})}x^{d_{v}(d_{u}+d_{v})}\\ &=&\sum\limits_{uv\in E_{1}(OX_{n})}x^{4(2+4)}+\sum\limits_{uv\in E_{2}(OX_{n})}x^{4(4+4)}\\ &=&\mid E_{2}(OX_{n})\mid x^{24}+\mid E_{2}(OX_{n})\mid x^{32}\\ &=& 12nx^{24s}+\left(18n^2-12n\right)\mid x^{32}. \end{eqnarray*} 4. \begin{eqnarray*} M_{a,b}(OX_{n},x)&=& \sum\limits_{uv\in E(OX_{n})}x^{ad_{u}+bd_{v}}\\ &=&\sum\limits_{uv\in E_{1}(OX_{n})}x^{2a+4b}+\sum\limits_{uv\in E_{2}(OX_{n})}x^{2a+4b}\\ &=&\mid E_{2}(OX_{n})\mid x^{2a+4b}+\mid E_{2}(OX_{n})\mid x^{2a+4b}\\ &=& 12nx^{(2a+4b)}+\left(18n^2-12n\right)x^{4(a+b)}. \end{eqnarray*} 5. \begin{eqnarray*} M'_{a,b}(OX_{n},x)&=& \sum\limits_{uv\in E(OX_{n})}x^{(d_{u}+a)(d_{v}+b)}\\ &=&\sum\limits_{uv\in E_{1}(OX_{n})}x^{(2+a)(4+b)}+\sum\limits_{uv\in E_{2}(OX_{n})}x^{(4+a)(4+b)}\\ &=&\mid E_{2}(OX_{n})\mid x^{(2+a)(4+b)}+\mid E_{2}(OX_{n})\mid x^{(4+a)(4+b)}\\ &=& 12nx^{(2+a)(4+b)}+\left(18n^2-12n\right)x^{(4+a)(4+b)}. \end{eqnarray*}

Theorem 2.2. Let \(OX_{n}\) be the Oxide network. Then,

1. \(Re ZG_{1}(OX_{n})=9n^{2}+3n\),
2. \(Re ZG_{2}(OX_{n})= 36n^{2}-8n\),
3. \(Re ZG_{3}(OX_{n})=2304n^{2}-960n\).

Proof.

1. \begin{eqnarray*} Re ZG_{1}(OX_{n})&=&\sum\limits_{uv\in E(OX_{n})}\frac{d_{u}+d_{v}}{d_{u}d_{v}}\\ &=&\frac{3}{4}(12n)+\frac{1}{2}(18n^{2}-12n).\\ &=& 9n^{2}-3n \end{eqnarray*} 2. \begin{eqnarray*} Re ZG_{2}(OX_{n})&=&\sum\limits_{uv\in E(OX_{n})}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}\\ &=&\frac{4}{3}(12n)+2(18n^{2}-12n).\\ &=& 36n^{2}-8n \end{eqnarray*} 3. \begin{eqnarray*} Re ZG_{3}(OX_{n})&=&\sum\limits_{uv\in E(OX_{n})}(d_{u}+d_{v})(d_{u}.d_{v})\\ &=&48(12n)+128(18n^{2}-12n).\\ &=&2304n^{2}-960n \end{eqnarray*}

Competing Interests

The authors declare that they have no competing interests.